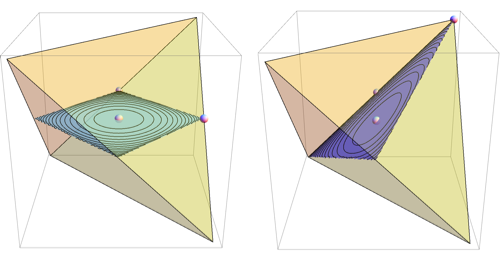
For 7d simplex, there are 49 such sections, and the most symmetrical one is the octahedron, which in the notebook below was found as the set of all 8 dimensional unit vectors with non-negative components orthogonal to (0, 3, 1, 0, -5, 0, 0, 1)
Here are all 49 distinct sections

Complete code is here.
An outline of how they were generated:
- Use Peter Shor's observation that there's a homomorphism between distinct sections and ways to assign 8 identical objects to vertices of the cube
- Find such permutations by breaking space of all permutations of 8 objects into orbits under action of group of cube rotations and taking one representative from each orbit, using PermutationGroup, GroupElements and Permute, implemented as nonEquivalentPermutations
- Use BooleanCounting function and SatisfiabilityInstances to find assignment of vertices to centroids consistent with given cube representation, implemented as findAssignment
- Use NullSpace, Reduce and FindInstances to find 0-dimensional intersections of hyperplane defining the section with some subset of 8 simplex cells, implemented as getExtremePoints and getCons
- Those point sets define vertices of the section, use SingularValueDecomposition to find vertex sets with distinct sets of four non-zero singular values, implemented as singularCert and distinctPoses
- Project distinct point sets onto hyperplane defining the section and use ComputationalGeometry`Methods`ConvexHull3D to get description of the shape in terms of polygons, implemented as getVerts3D and getPoly
Notebook
Interesting! I like this!
ReplyDeleteNice post. thank you for sharing with us. I think it would be effective for all which people are want to sale their home. I like this post indeed.
ReplyDeleteMathematica